Up: Experiment 8: INTERFERENCE
Previous: 8.3 The Index of Refraction of Air
In the above section the index of refraction of air was measured by slowly varying the
density of air along a fixed length of one beam path in the Michelson Interferometer.
That method obviously won't work with a solid substance, such as glass. Therefore,
in order to measure the index of refraction of glass, it's necessary to slowly vary the length of glass through which the interferometer beam passes. This experiment
introduces a technique for making such a measurement.
- Align the laser and interferometer in the Michelson mode.
- Place the rotating talbe between the beam splitter and movable mirror, perpendicular
to the optical path.
- Mount the glass plate on the magnetic backing of the rotational pointer.
- Position the pointer so that its ``0'' edge on the Vernier scale is lined up with the zero on the degree scale on the interferometer base.
- Remove the lens from in front of the laser. Hold the viewing screen between the glass plate and the movable mirror. If there is one bright dot and some secondary dots on the viewing screen, adjust the angle of the rotating table until there is one
- Replace the viewing screen and the lens and make any minor adjustments that are necessary to get a clear set of fringes on the viewing screen.
- Slowly rotate the table by moving the lever arm. Count the number of fringe transitions that occur as you rotate the table from 0 degrees to an angle
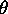 (where
(where
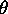 is at least 10 degrees).
is at least 10 degrees).
In principle, the method for calculating the index of refraction is relatively simple.
The light passes through a greater length of glass as the plate is rotated.
The general steps for measureing the index of refraction is as follows:
- Determine the change in the path length of the light beam as the glass plate is
rotated. Determine how much of the change in path length is through glass
 ,
and how much is through air,
,
and how much is through air, 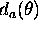 .
. - Relate the change in path length to your measured fringe transitions with the following equation:

where 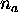 is the index of refraction of air (measured above),
is the index of refraction of air (measured above), 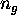 is the index of
refraction of glass (as yet unknown),
is the index of
refraction of glass (as yet unknown), 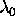 is the wavelength of the laser
in vacuum and N is the number of fringe transitions that you counted.
is the wavelength of the laser
in vacuum and N is the number of fringe transitions that you counted.
Carrying out this analysis (a bonus point for those who work this out before
the lab and show the T.A. the analysis), gives the following
result for the index of refraction in glass:

where t is the thickness of the glass plate.
Up: Experiment 8: INTERFERENCE
Previous: 8.3 The Index of Refraction of Air
![]()
![]() is the index of refraction of air (measured above),
is the index of refraction of air (measured above), ![]() is the index of
refraction of glass (as yet unknown),
is the index of
refraction of glass (as yet unknown), ![]() is the wavelength of the laser
in vacuum and N is the number of fringe transitions that you counted.
is the wavelength of the laser
in vacuum and N is the number of fringe transitions that you counted.
![]()